Aplicatii ale trigonometriei sferice in geodezie si astronomie

Aplicatii ale trigonometriei sferice in geodezie si astronomie

Partea I. - Geodezie.

131. Reducerea unui unghi la orizont.

Solutie generala.- Lasam OZ sa fie vertical observatorului la O; apoi, daca unghiul MON= a, NOZ = b, MOZ = c, se cere a se gasi proiectia MON pe un plan orizontal care trece prin O.

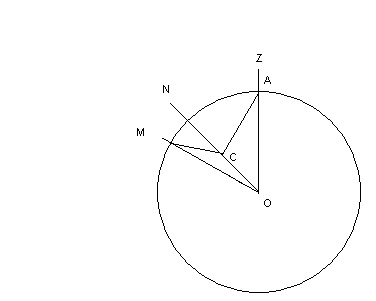
Sol.- Din O ca si centru, cu raza de 1unitate, descrie o sfera, trasand OZ, OM, ON prin A, B, C respectiv; apoi unghiul cautat A al triunghiului sferic BAC, care e dat de formula:

tan A =

A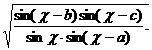plicatii ale Trigonometriei Sferice.

1. Solutia lui Legendre.- Aceasta solutie este aplicabila numai cand unghiurile de elevatie ale obiectelor M, N sunt foarte mici; asta este, cand b si c sunt ambele aproape de 90°. Depinde de urmatoarea lema : - Fiind dat un triunghiu sferic ABC, unghiul A₁ al triunghiului rectiliniu format de catre arcele sale (numit triunghiu chordal) e dat de ecuatia:

cos A₁ = sinb sinc + cos b cosc cos A. (458)

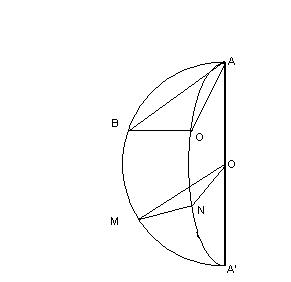
DEM. - Fie A'BC triunghiul colunar, M, N, punctele de mijloc ale arcelor A'B, A'C; si corzile AB, AC paralele cu razele PM, ON ale sferei. De unde

cos A₁ = cos MON = sin b sin c + cosb cosc cos A.

Cor. -If A= A-, atunci cos A₁= cos A + sin A aproximativ; si inlocuind in (458) pentru cosb cosc, sinb sinc, valorile cos² (b-c), sin² (b+c)-sin² (b-c), obtinem, dupa o reductie simpla,

= tgA sin² (b+c)- ctgA sin² (b-c). (459)

Fiind dat unghiul oblic continut intre 2 obiecte situate deasupra orizontului, pentru a afla unghiul orizontal corespunzator.

Geodezie. 145

Fie A pozitia observatorului, M, N obiectele de deasupra orizontului; fie o sfera de raza = 1 unitate, sa fie descrisa intersectand orizontul in A si intersectand dreptele AM, AN in punctele B, C. Desenati cercurile ABO, ACO; apoi daca H, H' indica ridicarea AM, AN deasupra orizontului, avem H= arc AB, H' =c, H'=b. Acum unghiul A al triunghiului sferic ABC in unghiul orizontal care corespunde unghiului oblic MAN. De unde, daca indica diferenta, avem (459)

= tg A sin²(b+c) - ctg A sin (b+c);

= tgA sin²(H+H') - ctgA sin (H-H'). (460)

In practica H,H' si sunt foarte mici. Deci aceasta formula poate fi inlocuita de urmatoarea, a lui Legendre:

= ² tg MAN- ²ctg MAN, (461)

O valoare aproximativa a diferentei dintre masurile circulare ale unghiurilor oblice si orizontale, care trebuie adaugate primei pentru a obtine ultima.

Aplicatii ale Trigonometriei Sferice.

132. Teorema lui Legendre.

Daca laturile unui triunghi sferic sunt mici in comparatie cu raza sferei, iar daca este contruit un triunghi plan ale carui sides sunt egale in lungime cu acelea ale unui triunghi sferic, atunci fiecare unghi al triunghiului sferic depaseste valoarea unghiului corespunzator in triunghiul plan cu 1/3 din excesul sferic. (by one-third of the spherical excees.

DEM.- Fie a, b, c lungimile laturilor triunghiului sferic, r raza sferei, atunci masurile arcelor (laturi) sunt

, ,

respectiv; de unde

cos A=;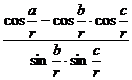

si, inlocuind cu cos , cos & c., valorile lor date in Pl. Trig. § 158, rezulta, neglijand puteri mai mari decat a 4-a parte a ,

cos A =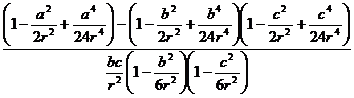

=

=

=

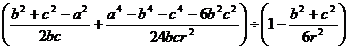
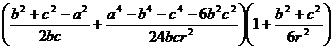
Geodezie.

Astfel daca , , indica unghiurile triunghiului plan, ale carui laturi sunt a, b, c, avem

cos A = cos a- aproximativ.

Acum, considerand A = a+ , avem cos A = cos a - sin a.

De unde = ,

S indicand aria triunghiului plan;

Similar, B - β = ,

C - =.

De unde = exces sferic;

exces sferic. (462)

133. Aria triunghiului sferic e aproximativ egala cu

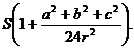(463)

DEM. tg

Dar tg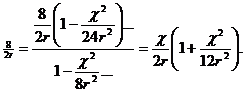

Deci

tg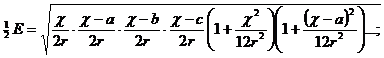

Aplicatii ale Trigonometriei Sferice.

Therefore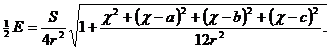

De unde 2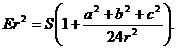

Cor.- Aria triunghiului sferic e egala cu aria triunghiului plan, daca omitem termenii de gradul 2 in . 134. Daca n reprezinta numarul patratelor intr-un exces sferic, aria triunghiului sferic la suprafata pamantului in picior patrat; atunci log n = - 9*3267737. - (General Roy.)

DEM.- Avem 2E=; . Si lungimile medii ale unui grad = 365155 picioare. Prin urmare = 365155; inlocuind valoarea lui r din aceasta ecuatie in valoarea lui , si scotand logaritmii, gasim

log n = - 9*3267737. (464)

Exercitii.-XXXV.

Unghiurile opuse laturilor unui triunghiu sferic la the pole circumferintei sunt respective dublii unghiurilor corespunzatoare arcelor triunghiului. respectively double of the corresponding angles of its chordal triangle.

Dovediti Teorema lui Legendre din formula pentru sin A, cosA, tan A, respectiv, in terms of the sides.

Daca raza pamantului ar fi de 4000 mile, cat ar fi in aria unui triunghi sferic a carui exces este de 1^o.

Daca A", B", C" sunt unghiurile chordal ale triunghiului polar al ABC, demonstrati

cosA" = sinA cos (s - a), &c. (465)

daca A'BC be the colunar lui ABC; demonstrati ca cosinusurile unghiurilor triunghiului chordal sunt respective egale cu respectively

cos cos E, sinb sin (C - E), sinc sin (B - E): (466)

Astronomie. 149

Daca R este circumferinta unui triunghiu sferic, A₁, B_1, C ₁ unghiurile triunghiului chordal; demontrati

sin A₁ = sin cosec R, sin B₁ = sinb cosec R, sin C₁ = sinc cosec R. (467)

7. Demontrati sin A₁ : sin (B₁ - C₁) : sin A : sin (B - C). (468)

8. Demonstrati propozitia din § 132 din ecuatia (351).

9. Demonstrati E = (tg tgb) sin C - (tg tgb)² sin 2C. (469)

[Folositi valorile lui tg E rezultate din ecuatiile (351), (356).]

Aratati ca in fiecare caz solutiile triunghiului sferic, exceptand unde cele 3 unghiuri sunt date, ca teorema lui Legendre poate fi folosita pentru o solutie aproximativa.

Partea II.-Astronomie.

135. Definitii Astronomice.

Daca PHNR reprezinta meridianul oricarui loc, generat pentru a intalni sfera cereasca, P - Polul Nord, O - Polul Sud

Cerurile, HR orizontul, EQ ecuatorul, Z zenitul; apoi, pentru un loc al carui zenith este Z, QZ e latitudinea.

Aplicatii ale Trigonometriei Sferice.

Din moment ce QZ este clar egal cu PR, PR e egal cu latitudinea; dar PR e ridicarea polului deasupra orizontului. Asadar cota polului e egala cu latitudinea.

Din nou, daca S ar fi orice corp ceresc, ca soarele sau o stea, pozitia lui e determinata de oricare dintre cele 4 sisteme de coordonate dupa cum urmeaza:

1^o. Marea rotatie ZST trecand prin zenit si S, si intalnint orizontul in T, e numita orbita verticala al S. The are? HT, masurat de la punctul sudic al orizontului, sau echivalentul sau, unghiul HZT, care e numit azimut, si ST altitudinea. HT, ST sunt coordonatele sferice ale stelei S; ZS in distanta sa zenitala, si arcul RT azimutul nordic.

2^o. Join SP, and produce to meet the equator in K. The arcs QK, KS form the second system

of spherical co-ordinates; QK, or its equal the angle ZPS, is called the hour angle of S, and KS the declination. The declination is positive when S is north of the equator, and negative when south. The great circle PSK is called the declination circle, and PS the polar distance of S.

3^o. The great circle which the centre of the sun, seen from the centre of the earth, appears to describe annually among the stars is called the ecliptic; and its inclination to the equator, which is nearly 23^o, the obliquity of the elliptic. This points of intersection of equator and ecliptic are called the equinoxes- one the vernal equinox (called also the first point of Aries), and the vernal equinox (the first point of Libra). If ν denote the first point of Aries, then νK is called the right ascension, and KS the declination of the star; νK, KS are the third system of spherical co-ordinates of S. The right ascension is counted eastward, from 0 to 360^o.

4^o. From S draw a great circle Sσ perpendicular to the ecliptic; then νσ, σS are the fourth system of spherical zo-ordinates of S, and are called respectively its longitude and

Astronomie 151

latitude. The longitude is reekoned eastward, from 0 to 360^o. The latitude is positive when north, and negative when south.

If the small circle, M, M' passing through S, and parallel to the equator, represent the apparent diurnal motion of the sun or other heavenly body (the declination being supposed constant), it is evident he will be rising or setting at A (according as the eastern of the western hemisphere is represented by the diagram), He will be east or west at , will be at B at 6 o'clock, morning or evening, will be at noon at M, and at midnight at M'.

The foregoing definitions and diagram will enable us to solve several astronomical problems of an elementary character, such as the following:

1^o. To find the time of rising or setting of a known body.

Consider the spherical triangle APR. We have

cos RPA = tan RP. Cot AP.

Hence, denoting the hour angle APZ by t, the latitude by , and the declination by δ, we have

cos t = - tan tan δ. (470)

And the hour angle being known, the time may be found. In the case of the sun, the formula (470) gives the time from sunrise to noon, and hence the length of the day.

2^o. being given the declination and the latitude, to find the azimuth from the north at rising.

Let A denote the required azimuth, then A = AR. Hence, from the triangle ARP, we have

sin δ = cos * cos A. (471)

3^o. Being given the hour angle and declination of a star, to find the azimuth and altitude.

Aplicatii ale Trigonometriei Sferice.

Let Z denote the zenith distance ZS, A the azimuth from the north, p the angle ZSP at the star; then, by Delambre's Analogies,

cos. (472)

cos. (473)

sin. (474)

sin. (475)

Hence, when t, δ, are given; that is, the hour angle and declination of a heavenly body, and the latitude of the observer, z, p, A can be found. In a similar manner may be solved the converse problem:-Given the azimuth and altitude, to find the hour angle and the declination.

4^o. If denote the altitude of the sun at 6 o'clock, and the altitude when east or west; then

(476)

sin a' = sin δ sin . (477)

Exercises.- XXXVI.

In latitude 45^o N., prove that the shadow at noon of a vertical object is three times as long when the sun's declinations is 15^o S. as when it is 15^o N.

The altitude of a star when due east was 20 ^o, and it rose EbN; required the latitude.

Given the sun's longitude, to find his right ascension and declination.

Let denote the right ascension, δ the declination, the obliquity of the ecliptic. Now let S denote the sun's place in the ecliptic γS. Trasati

153

SD perpendicular cu γD, ecuatorul; deci daca λ denote the longitude, we have the triangle γSD,

tg α = cos tg λ. (478)

sin δ = sin sin λ (479)

Given the azimuth of the sun at setting, and at 6 o'clock, find the sun's declination, and the latitude.

If the sin's declination be 15^o N., and length of day four hours, prove tan = sin 60^o tan 75^o.

prove that cos (480)

Given the sun's declination and the latitude, show how to find the time when he is due east.

If the sun rise N.E. in latitude , prove that

Cot hour angle at sunrise = - sin .

Given the meridian altitude and altitude when east, find the latitude and the declination.

Given the roght ascension and the declination of a star S, to find its latitude and longitude.

Let D,L be the equator and the elliptic, S the star, SD, SL perpendicular to D, L; then, if α be the right ascension, δ the declination, the latitude, and λ the longitude of S, denoting the angle SD by, from the right-angled triangles S, SL, we get

ctg = sin α ctg , (481)

The first of these equations determines , and the others and .

Being given the latitudes and longitudes of two places on the earth considered as a perfect sphere, to find the distance between them.

This is evidently a case of § 66, viz., when two sides and the contained angle are given, to find the third side.

Find the latitude, being given the declination, and the interval between the time the sun is west and sunset.

If the latitudes and longitudes of two places on the earth be given, show how to find the highest latitude attained by a ship in sailing along a great circle from one place to the other.

Being given the latitudes and longitudes of two places, find the sun's declination when he is on the horizon of both at the same instant.

If the difference between the lengths of the longest and the shortest day at a given place be six hours, find the latitude.

If two stars rise together at two places, prove that the places will have the same latitude; and if they rise together at one place, and set together at the other, the places will have equal latitudes of opposite names.

If p₁, p₂ be the radii vectors of two planets which revolve in circular orbits, prove, if when they appear stationary to one another, the cotangent of P₂' elongation, seen from P₁, be tan , that

2 p₁ = p₂ tg * tg . (482)

If be the declination of a heavenly body, which in its diurnal motion passes in the minimum time from one to another of two parallels of altitude, whose zenith distances are Z, Z', prove that

sin δ = sin lat. (483)

If be the latitude, the obliquity of the ecliptic, prove that if the lengths of the shadow of an upright rod at noon on the longest and the shortest days be as 1: n,

sin 2: sin 2: n + 1 : n - 1. (484)